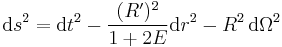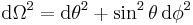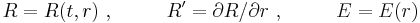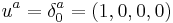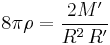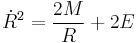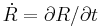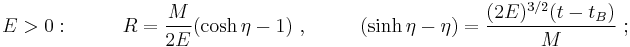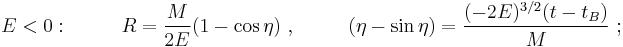Lemaître–Tolman metric

The Lemaître–Tolman metric is the spherically symmetric dust solution of Einstein's field equations was first found by Lemaître in 1933 and then Tolman in 1934. It was later investigated by Bondi in 1947. This solution describes a spherical cloud of dust (finite or infinite) that is expanding or collapsing under gravity. It is also known as the Lemaître-Tolman-Bondi metric and the Tolman metric.

The metric is:

$\mathrm{d}s^{2} = \mathrm{d}t^2 - \frac{(R')^2}{1 %2B 2 E} \mathrm{d}r^2 - R^2 \, \mathrm{d}\Omega^2$

where:

$\mathrm{d}\Omega^2 = \mathrm{d}\theta^2 %2B \sin^2\theta \, \mathrm{d}\phi^2$

$R = R(t,r)~,~~~~~~~~ R' = \partial R / \partial r~,~~~~~~~~ E = E(r)$

The matter is comoving, which means its 4-velocity is:

$u^a = \delta^a_0 = (1, 0, 0, 0)$

so the spatial coordinates 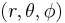$(r, \theta, \phi)$ are attached to the particles of dust.

The pressure is zero (hence dust), the density is

$8 \pi \rho = \frac{2 M'}{R^2 \, R'}$

and the evolution equation is

$\dot{R}^2 = \frac{2 M}{R} %2B 2 E$

where

$\dot{R} = \partial R / \partial t$

The evolution equation has three solutions, depending on the sign of $E$ ,

$E > 0:~~~~~~~~ R = \frac{M}{2 E} (\cosh\eta - 1)~,~~~~~~~~ (\sinh\eta - \eta) = \frac{(2 E)^{3/2} (t - t_B)}{M}~;$

$E = 0:~~~~~~~~ R = \left( \frac{9 M (t - t_B)^2}{2} \right)^{1/3}~;$

$E < 0:~~~~~~~~ R = \frac{M}{2 E} (1 - \cos\eta)~,~~~~~~~~ (\eta - \sin\eta) = \frac{(-2 E)^{3/2} (t - t_B)}{M}~;$

which are known as hyperbolic, parabolic, and elliptic evolutions respectively.

The meanings of the three arbitrary functions, which depend on $r$ only, are:

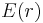$E(r)$ - both a local geometry parameter, and the energy per unit mass of the dust particles at comoving coordinate radius $r$ ,
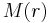$M(r)$ - the gravitational mass within the comoving sphere at radius $r$ ,
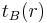$t_B(r)$ - the time of the big bang for worldlines at radius $r$ .

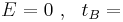Special cases are the Schwarzschild metric in geodesic coordinates $M =$ constant, and the Friedmann–Lemaître–Robertson–Walker metric, e.g. $E = 0~,~~ t_B =$ constant for the flat case.

References

Bondi, Hermann (1947). "Spherically symmetrical models in general relativity". Monthly Notices of the Royal Astronomical Society 107: 410. Bibcode 1947MNRAS.107..410B.
Krasinski, A., Inhomogeneous Cosmological Models, (1997) Cambridge UP, ISBN 0 521 48180 5
Lemaitre, G., Ann. Soc. Sci. Bruxelles, A53, 51 (1933).
Tolman, Richard C. (1934). "Effect of Inhomogeneity on Cosmological Models". Proc. Natl. Acad. Sci. (National Academy of Sciences of the USA) 20 (3): 169. PMC 1076370. PMID 16587869. Archived from the original on 2011-01-27. http://www.webcitation.org/5w35fIoge. Retrieved 2011-01-27.